Applications of the two-variable expansion procedure to problems in celestial mechanics

A P P L I C A T I O N O F T H E T WO-VAHABLE EXPANSION P R O C E D U R E TO P R O B L E M S IN C E L E S T I A L MECHANICS

T h e s i s b y J e r r y L e e S i m m o n s

In P a r t i a l F u l f i l l m e n t of t h e R e q u i r e m e n t s F o r t h e D e g r e e of

A e r o n a u t i c a l E n g i n e e r

C a l i f o r n i a I n s t i t u t e of T e c h n o l o g y P a s a d e n a , C a l i f o r n i a

ABSTRACT

This work i l l u s t r a t e s the application of the t w o - v a r i a b l e

expansion p r o c e d u r e of r e f e r e n c e 1 to the solution of two r e p r e s e n t a t i v e p r o b l e m s in c e l e s t i a l m e c h a n i c s .

The expansion p r o c e d u r e i s applied f i r s t to the p r o b l e m of a e r o - dynamic p e r t u r b a t i o n s of a s a t e l l i t e o r b i t , The c a s e of p l a n a r motion i s c o n s i d e r e d with both lift and d r a g p e r t u r b a t i o n s acting on the s a t e l l i t e . A simplified model of t h e e a r t h i s u s e d , but the motion i s expected t o exhibit a s i m i l a r qualitative behavior i n the m o r e g e n e r a l c a s e . It i s found that the effect of d r a g c a u s e s the s a t e l l i t e to s p i r a l toward the c e n t e r of a t t r a c t i o n while the o r b i t i s tending to become c i r c u l a r . The effect of lift, to the o r d e r computed, i s f e l t only by a slow advance of the a p s e .

The second application of t h e expansion p r o c e d u r e i s to t h e

p r o b l e m of third-body p e r t u r b a t i o n s of a s a t e l l i t e o r b i t . A s p e c i a l c a s e of t h e r e s t r i c t e d three-body p r o b l e m is u s e d in which the plane of the

s a t e l l i t e ' s o r b i t i s coincident with the o r b i t a l plane of the two l a r g e r bodies, The two-variable expansion i s applied to approximate

ACKNOWLEDGMENTS

The author would like t o thank D r . 'W. W. Royce f o r his suggestion of the p r o b l e m of a e r o d y n a m i c p e r t u r b a t i o n s and f o r his valuable guidance during the a c a d e m i c y e a r 1960-61,

The author i s v e r y deeply indebted t o D r . J . K O Kevorkian who s u g g e s t e d the p r o b l e m of third-body p e r t u r b a t i o n s and

provided e x t r e m e l y valuable guidance and e n c o u r a g e m e n t nece s s a r y f o r the solution of both the a e r o d y n a m i c and third-body p e r t u r b a t i o n p r o b l e m s .

T A B L E O F CONTENTS

INTRODUCTION

P a g e 1 AERODYNAMIC PERTURBATIONS O F A S A T E L L I T E

ORBIT

2. 1 F o r m u l a t i o n of t h e P r o b l e m

2.2 Equations of Motion and I n i t i a l Conditions

2 . 3 The T w o - V a r i a b l e E x p a n s i o n 2 . 4 Solution f o r t h e K e p l e r i a n O r b i t

2.5 Solution f o r the F i r s t - O r d e r P e r t u r b a t i o n s 2 . 6 S u m m a r y a n d D i s c u s s i o n of R e s u l t s

111. THIRD-BODY PERTURBATION O F A S A T E L L I T E ORBIT

3. 1 F o r m u l a t i o n of t h e P r o b l e m 3 . 2 E q u a t i o n s of Motion

3 . 3 The T w o - V a r i a b l e E x p a n s i o n 3 . 4 I n i t i a l Conditions

3 . 5 Solution f o r the K e p l e r i a n O r b i t

3 . 6 Solution f o r t h e F i r s t - O r d e r P e r t u r b a t i o n s 3 . 7 Solution f o r the S e c o n d - O r d e r P e r t u r b a t i o n s 3 . 8 C o m p a r i s o n of t h e R e s u l t s with R e f e r e n c e 2

3 . 9

S u m m a r y

I. INTRODUCTION

This work i l l u s t r a t e s the application of the two-variable expansion p r o c e d u r e of r e f e r e n c e 1 to the solution of the following two r e p r e s e n t a t i v e p r o b l e m s i n c e l e s t i a l m e c h a n i c s .

1) Aerodynamic perturbations* of a s a t e l l i t e o r b i t . 2 ) Third-body p e r t u r b a t i o n of a s a t e l l i t e o r b i t .

In both of the above p r o b l e m s , c e r t a i n simplifying a s s u m p t i o n s have been made i n formulating the physical p r o b l e m i n o r d e r t o e a s i l y e s t a b l i s h the b a s i c i d e a s of the technique and provide the n e c e s s a r y p r e l i m i n a r y s t e p i n t h e solution of the m o r e g e n e r a l c a s e s .

Although i n both of t h e s e p r o b l e m s the motion i s K e p l e r i a n i n the a b s e n c e of the perturbing f o r c e s , the n a t u r e of the p e r t u r b a t i o n s and the behavior of t h e solutions a r e quite different i n e a c h c a s e .

In p r o b l e m f l ) , the p e r t u r b a t i o n due t o a e r o d y n a m i c d r a g i s d i s s i p a t i v e ; and t h i s c a u s e s the s e m i - m a j o r a x i s of the osculating e l l i p s e t o d e c r e a s e slowly with t i m e . The two-variable expansion p r o c e d u r e was specifically developed f o r p r o b l e m s with d i s s i p a t i v e p e r t u r b a t i o n s w h e r e other methods, s u c h a s the method of v a r i a t i o n of e l e m e n t s , f a i l t o provide uniformly valid approximations f o r l a r g e t i m e s .

p o s s e s s a c o n s e r v a t i o n l a w (the J a c o b i i n t e g r a l ) . As a r e s u l t , the e l e m e n t s of the osculating e l l i p s e undergo bounded v a r i a t i o n s i n s o m e p r e c e s sing c o o r d i n a t e s y s t e m .

11. AERODYNAMIC PERTURBATIONS O F A S A T E L L I T E ORBIT

2.1 F o r mulation of the P r o b l e m

----

The p r o b l e m of d r a g p e r t u r b a t i o n s of a s a t e l l i t e o r b i t h a s been solved i n r e f e r e n c e 1 by the t w o - v a r i a b l e expansion p r o c e d u r e . In t h i s s e c t i o n of the p r e s e n t work, the p e r t u r b a t i o n s of a s a t e l l i t e o r b i t due to a e r o d y n a m i c lift and d r a g will be d e t e r m i n e d by using the two - v a r i a b l e expansion p r o c e d u r e .

The physical m o d e l to be u s e d will be the s a m e a s that i n r e f e r e n c e 1. A s a t e l l i t e i s a s s u m e d to move i n a p l a n a r o r b i t about a homogeneous, s p h e r i c a l , and non-rotating e a r t h s u r r o u n d e d by a constant d e n s i t y a t m o s p h e r e , It i s a l s o a s s u m e d that no o t h e r c e l e s t i a l bodies influence t h e motion of the s a t e l l i t e , The d r a g coefficient and l i f t - d r a g r a t i o of the s a t e l l i t e a r e taken to be c o n s t a n t s . This physical model a d m i t t e d l y d e p a r t s c o n s i d e r a b l y f r o m r e a l i s m , and i s p r e s e n t e d only t o i l l u s t r a t e the qualitative behavior of the motion, The m o r e r e a l i s t i c model with a v a r i a b l e density, and a e r o d y n a m i c coefficients depending on Mach n u m b e r and angle of a t t a c k can be t r e a t e d by t h i s method a t the c o s t of c o n s i d e r a b l e a l g e b r a i c complication,

2.2 Equation of Motion and I n i t i a l Conditions

-

where

C = d r a g coefficient of the s a t e l l i t e D

G = u n i v e r s a l gravitation constant M = m a s s of the e a r t h

m = m a s s of the s a t e l l i t e

n = l i f t - d r a g r a t i o of the s a t e l l i t e

r

=

r a d i a l d i s t a n c e f r o m c e n t e r of e a r t h t o s a t e l l i t e

S

= r e f e r e n c e a r e a of the s a t e l l i t e t = t i m e

p = a t m o s p h e r i c d e n s i t y

ep

= t r u e anomally

If the following d i m e n s i o n l e s s quantities a r e chosen

where

R

= i n i t i a l r a d i a l d i s t a n c e of the s a t e l l i t e

T = p e r i o d of the K e p l e r i a n o r b i t c o r r e s p o n d i n g t o the i n i t i a l conditions =

71a3

/ G M

(2. l b )

c = a e r o d y n a m i c p a r a m e t e r ( r a t i o of d r a g t o c e n t r i f u g a l f o r c e initially)

*

2 ::: 2 1

/

2

2 d r dcp -

r d +

- - -

-

-,

[*?

t

$1

[(:$I

+

r*2

[312]

( 2 0 2 b ) d t *2 dt:; dt*

:k :::

These equations a r e next t r a n s f o r m e d ' s 9 t h a t u ( = l / r ) and t become the dependent v a r i a b l e s and

cp

the independent v a r i a b l e . Hence,

The following i n i t i a l conditions a r e adopted:

2 . 3 The Two-Variable Expansion

A s indicated i n r e f e r e n c e 1, the decay of the o r b i t due t o d r a g will be exhibited i n the solution by the behavior of u with r e s p e c t to the slow v a r i a b l e = ecp; and i t will be r e q u i r e d that u be a bounded function of cp f o r fixed

$.

Equations (2.3) will be solved by the two-variable

:::

Then

..

=

u;O)i

c ( U U t 0 ( c 2 ) dcp

::: 2::: 2

Equations (2.7) give the solution f o r G = 0, w h e r e the motion of the s a t e l l i t e i s d e s c r i b e d by a K e p l e r i a n e l l i p t i c a l o r b i t . Equations (2.8) give the solutions f o r the f i r s t - o r d e r p e r t u r b a t i o n s due t o a e r o d y n a m i c f o r c e s .

2.4 Solution f o r the K e p l e r i a n O r b i t

I n t e g r a t i o n of equation c2.7b) g i v e s

With this r e s u l t , the solution of equation ( 2 , 7 a ) i s

w h e r e

2

f = 1

2 !by definition)

a(1-e )

a = at@) = d i m e n s i o n l e s s s e m i - m a j o r a x i s e = eCQ) = e c c e n t r i c i t y

=

PC$)

= longitude of the a p s e

Using equation (2.9b), equation [2,9a) m a y be i n t e g r a t e d t o give

The q u a n t i t i e s a, e,

P

and T which c o r r e s p o n d t o t h e f o u r i n t e g r a t i o n

c o n s t a n t s f o r K e p l e r i a n motion, a r e now functions of and will be evaluated subsequently,

2.5 Solution f o r the F i r s t - O r d e r P e r i u r b a t i o n s

v

Now l e t

s o that i n t e g r a t i o n of equation (2.11) g i v e s

The quantity P m a y be obtained by d i r e c t i n t e g r a t i o n of equation (2.12). The i n t e r e s t of t h i s work i s i n o r b i t s of s m a l l e c c e n t r i c i t y , s o that only t e r m s which a r e l i n e a r i n e will be r e t a i n e d , Thus

1

P =

- -

[rp

+

e sin(ep-a) t ne cos[rp-p)] t 0 ( e 2 )

f (2,lS)

Then equation ( 2 , 8 a ) b e c o m e s

s o t h a t

In o r d e r that ~ ' l ) be a bounded function of

rp,

the t e r m s p r o - portional to cp, sin(y-p), and cos(cp-@) in equation (2,17) m u s t vanish, Hence:

The solutions of equations (2.18) c o r r e c t to o r d e r [e) and satisfying the i n i t i a l conditions (2.4) a r e :

The quantity e i s the initial e c c e n t r i c i t y and i s a s s u m e d to be s m a l l 0

c o m p a r e d to unity.

Equation f2.17) then b e c o m e s

w h e r e A and B m u s t be d e t e r m i n e d by the boundedness r e q u i r e m e n t on

u ' ~ ) ~

I n s e r t i o n of equatinn (2.21) into equation (2.13) yields

5

-

5 _- 5 _- 5

2 2 2

-(Za7B-5a en+lOa cos(9-p)-3a eA sinZ(cp-P)-3a e B c o s 2(?-P)

and i n t e g r a t i o n gives

In t h i s equation, the t e r m l i n e a r i n cp m a y be eliminated by requiring that

5 5 5

f r o m t h e boundedness r e q u i r e m e n t o n IJ12). In equation (2.24), the t e r m q u a d r a t i c i n cp cannot be eliminated. It i s t h e r e f o r e c h a r a c t e r - i s t i c of t h i s p r o b l e m that the t i m e i s a n unbounded function of cp.

2.6 S u m m a r y and D i s c u s s i o n of R e s u l t s

The r e s u l t of the preceding a n a l y s i s m a y b e s u m m a r i z e d a s follows:

1 2

=

-

[1-e cos(cp-P) t E. [A sin(cg-P)tB costcp-P)+

2fg-nl t O[e

) (2.26a) a

where

7 = 7(G) [given by equation 2.25)

explicitly d e t e r m i n e d by r e q u i r e m e n t s on

uC2)

and t"'. The

dominant behavior of the motion i s a l r e a d y exhibited by the f o r m of the functions a, e and

p.

The e f f e c t of d r a g i s to c a u s e the s e m i - m a j o r a x i s and

e c c e n t r i c i t y to tend t o z e r o monotonically a s i n c r e a s e s . Thus the o r b i t g r a d u a l l y s p i r a l s toward the c e n t e r of a t t r a c t i o n and tends t o become c i r c u l a r . This behavior justifies the o m i s s i o n of q u a d r a t i c t e r m s i n e i n the solution i f the i n i t i a l e c c e n t r i c i t y i s sufficiently s m a l l . The effect of lift i s , t o t h i s o r d e r , only f e l t by a slow advance of t h e a p s e a s shown by equation f 2 . 2 7 ~ ) . In o r d e r to evaluate the p e r i o d of t h i s s p i r a l i n g motion, i t i s n e c e s s a r y t o c a r r y the compu- tations one s t e p f u r t h e r and c a l c u l a t e T which depends on

B

and g.

111. THIRD-BODY PERTURBATION O F A S A T E L L I T E ORBIT

3 . 1 -- F o r m u l a t i o n of the P r o b l e m

This p r o b l e m h a s been solved i n r e f e r e n c e 2 b y a method

I

s i m i l a r t o t h a t of P o i n c a r e f o r periodic s y s t e m s . In the method of r e f e r e n c e 2, v a r i o u s coordinate t r a n s f o r m a t i o n s a r e rnade i n o r d e r to account f o r the p e r t u r b a t i o n s i n the s a t e l l i t e o r b i t due to the s u n ' s g r a v i t a t i o n a l field. In the p r e s e n t work, i t will be shown t h a t the two- v a r i a b l e expansion p r o c e d u r e provides a m o r e s y s t e m a t i c a p p r o a c h

requiring no p r i o r knowledge of the solution.

The r e s t r i c t e d t h r e e - b o d y f o r m u l a t i o n will b e u s e d i n which the s u n and planet a r e a s s u m e d t o move i n c i r c u l a r o r b i t s about t h e i r common c e n t e r of m a s s . The m a s s of the planet i s a s s u m e d to be much s m a l l e r t h a n the m a s s of t h e s u n . The analysis of the p r e s e n t work will f u r t h e r be r e s t r i c t e d t o t h e c a s e w h e r e the plane of t h e s a t e l l i t e ' s o r b i t coincides with the o r b i t a l plane of the sun-planet s y s t e m .

3.2 Equations of Motion

The dimensional equations of motion of the s a t e l l i t e with

r e s p e c t t o a coordinate s y s t e m c e n t e r e d a t the m a s s - c e n t e r of the sun- planet s y s t e m and revolving with the planet a r e : [ s e e f i g u r e 1)

where

G = u n i v e r s a l gravitation constant m = m a s s of the planet

P

m = m a s s of the s u n S

r = d i s t a n c e f r o m s a t e l l i t e to planet =

P

rS = d i s t a n c e f r o m s a t e l l i t e t o s u n =

7

(gS-5)

+

_'7

-

t = t i m e

o .= a n g u l a r velocity of planet with r e s p e c t t o m a s s - c e n t e r

F i g u r e 1

-

Satellite

Sun

7

D

It i s shown i n r e f e r e n c e 2 that f o r a s a t e l l i t e i n c l o s e p r o x i m i t y to the planet, the following a p p r o x i m a t e equations can be d e r i v e d f r o m equations ( 3 .I):

3 e2 dt dS L.

+ Z

Iz]

s i n 2 6 - s c o s 2cp

3

t of-e4)

3.3 The Two-Variable Expansion _----

--

JI

Since rq". a p p e a r s explicitly i n equations (3.3), i t will be u s e d a s the f a s t v a r i a b l e . In o r d e r to allow f o r slight v a r i a t i o n s i n the a r g u m e n t s of the t r i g o n o m e t r i c t e r m s ap2earing in the solution, the

2 :x

slow v a r i a b l e will be chosen i n the f o r m = s [ l t a e t O { e ) ]qo

,

where a i s a n unknown constant t o be d e t e r m i n e d by r e q u i r i n g t h e solution to be bounded with r e s p e c t t o F . The following expansions a r e then a s s u m e d :

JI

-4.

-

S = s(cp

,

~9 (3.4a)

*

*

2 (2)

*

t - t ₂

[

[ 0 ) ) 2 [S/')sin 2cp

"

s

(0) c o s 2.

:%I

(1) (0) (0)

(0) t(2) (1) _{(0) + 2 S1 +S2} t 2 t [ 0 ) [ ~ j ~ ) t S ~ ) t a s j 0 ) ) t 2s1

(

i t 2 t a t 2

(

) [

i t 2

1

3

(0) (0) (1) (0) -2 t :x

= -4s 1

r

1 (tl i t 2

)

[

~ " ) ) 2 ( ~ ~ ) t ~ ~ ) ) t

;

sf0)

[t!')

1

s i n 2cp (3.7b)

3.4 I n i t i a l Conditions

The i n i t i a l conditions f o r e q u a t i o n s f3.5), (3.6) a n d ( 3 , 7 ) will b e c h o s e n s o t h a t t h e r e s u l t s of t h i s w o r k will be c o r n p a r a b l e t o t h o s e of r e f e r e n c e 2. In o r d e r t o do t h i s , i t i s n e c e s s a r y t o d e v e l o p a

:%

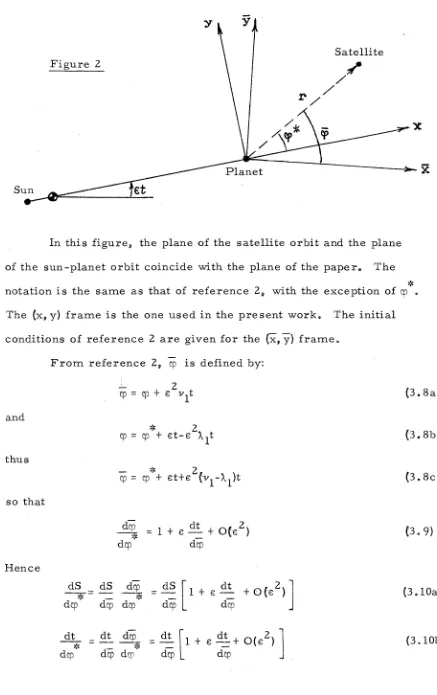
F i g u r e 2

In t h i s f i g u r e , the plane of the s a t e l l i t e o r b i t and the plane of the sun-planet o r b i t coincide with the plane of the p a p e r , The

rlr

notation i s t h e s a m e a s that of r e f e r e n c e 2, with the exception of cp*'.

The (x, y) f r a m e i s the one used i n the p r e s e n t work. The i n i t i a l

- -

conditions of r e f e r e n c e 2 a r e given f o r the (x, y) f r a m e . F r o m r e f e r e n c e 2, i s defined by:

and

thus

s o that

[image:21.532.52.489.49.744.2]

The i n i t i a l conditions a t = 0 a s given i n r e f e r e n c e 2 a r e :

Hence, t h e i n i t i a l conditions f o r the p r e s e n t f o r m u l a t i o n a r e :

where

a O = d i m e n s i o n l e s s i n i t i a l s e m i - m a j o r a x i s

e o

= i n i t i a l e c c e n t r i c i t y

Finally, the expansions f o r S and t given by (3 - 4 ) and f o r

.I. .I.

d ~ / d c p - ~ and dt~dcp"' given below dS

--

=

si0)

+

i ( S p + S y i )

+

0 ( c 2 )

_(3.13a)

i m p o s e the following eight initial conditions on equations C3.5) and (3.6):

3.5 Solutinn f o r the Keplerian Orbit

7-

-

The solution of equations (3.5) i s a g a i n

where the c o n s e r v a t i o n r e l a t i o n

has been used. The function f i s defined a s

The quantities e, a,

P

and T a r e functions of to b e d e t e r m i n e d by el)

3.6 Solution f o r the F i r s t - O r d e r P e r t u r b a t i o n s

--

Equation (3.6b) m a y now be w r i t t e n a s :

and c a n be d i r e c t l y i n t e g r a t e d t o the f o r m :

I n s e r t i o n of (3.19) into (3.6a) yields:

Expanding equation ( 3 , 2 0 ) i n t e r m s of e and retaining t e r m s to o r d e r f e ) , gives:

In o r d e r that ~ ( l ) be a bounded function of cpL, the coefficients

-:c :x o

The solutions of t h e s e equations a r e

1

f = constant = f -

0 -

l;jm

t a o U - e o e = constant = e

0 312,

P

= - a o cp + O ( e )

The solution f o r s(') i s then

w h e r e A and B a r e functions of to be d e t e r m i n e d . Equation t3.19) now b e c o m e s

5

-

-

5

- ( 3 g a o 2 t 3 e o a ~ ~

+

*)

-3e a [A s i n ~ ( m * - ~ ) + B c o s 2(cp*-p)

1

(3.25)

d 5 0 0

and c a n b e i n t e g r a t e d to:

-

-

2

*

- ( 3 g a o 2 t 3 e o a o 2 B t *)eplt e o a o [A cos2(rp - p ) - B s i n ~ ( c p ' " - ~ ) ]

+

h($)

d G

.I.

In t h i s e x p r e s s i o n , the t e r m l i n e a r i n cp i s e l i m i n a t e d by r e q u i r i n g t h a t 5

3.7 Solution f o r the Second-Orde r P e r t u r b a t i o n s

-

-

With the u s e of equations (3.16) and (3.19), equation (3.7b) may be written i n the f o r m :

2f oS

--

a

[

t t t tat3))

f 2 ) 3i0(s(l)\2 t II;S(~) z g s ( l )

a

- 7 -

( s ( o ) ) 2

( s ( o ) , 3 t ~ -

3

f:

.I.

-

-

- --

2 s i n 2cp-'*

( d o )

l4

Now l e t

2 3

Then integration of equation (3.28) gives

where

Q

i s given by

-3e0 .,. -,. 3 .b -,. e

Q = -cos(ip

t p )

-

--

cos 2cp

- -

C O S ( ~ $ - ~ )

Equations t3.3 0) and (3.31) a r e i n s e r t e d i n ( 3 , 7 a ) to give:

0

*

3

*

2eo

1\ f O 4

4 .

* -- _{4 COS("}

+p)

_-

-7

_{cos 2cp}

-

-

_{c o s ( 3 0}

-P)

+

_2f0k

+

--

0 2 f 0

sf

O) 2

2

*

3

f o

*

t ; f o (s(0)

/

4 sin 2 q

- -

c o s 2cp jS(0) ) 3

Evaluating a l l t e r m s i n t h e above equation and r e t a i n i n g t e r m s to o r d e r {e), y i e l d s

6Be0

*

3

*

2 1 2Be0

-

cos 2 ( ~

-p)-

-

4 c o s 2cp t ZfOk t g

-

---- 4

- --

3 (3.33)

*

H e r e i t should be noted that cp was c h o s e n a s the f a s t v a r i a b l e s o that the t r i g o n o m e t r i c f o r c i n g functions of equations (3.7) would not

1 2

i n t r o d u c e t e r m s of o r d e r

(x),

( E ) , o r ( C ) into t h e solution of equation (3.33). I t m a y be s a i d i n g e n e r a l that i f the two-variable expansion i s applied to d i f f e r e n t i a l equations i n which the a r g u m e n t s of the trigono-

n

m e t r i c f o r c i n g functions have the f o r m (1-s )rp, then the f a s t v a r i a b l e t

should be c h o s e n a s cp ={l-cn)rp

.

With the u s e of f a m i l i a r t r i g o n o m e t r i c i d e n t i t i e s , equation (3.33) m a y be r e w r i t t e n a s :

-

2dA 4.

)

]

0 0

2

*

t 7 e O a 0 2 t 6 e g a t Z a e O a O c o s p _{0 0 0} c o s c 5 e a cos(3,y

-a)

-

*

-

2

*

2

*

- 6 e o a o 2 A s i n 2(rp -PI-6Beoao c o s 2 ( 9 - P ) - 3 a 0 cos 2rp t 2 f O k

*b

Since S") m u s t be a bounded function of

6,

the following conditions a r e i m p o s e d on equation 33.34):

-

dg = 0 s o that g = g = c o n s t a n t

dG 0

and

-

*

s i n ~t 2dA t 7eoao2t6e g a e O a o 2

)

C O S = O

1

d B dA

-

c o s p -

-

2 + 3 e g a

dc7; dG 0 0 0

The solution of the above l i n e a r s y s t e m f o r A and

B

i s :

w h e r e A and B a r e c o n s t a n t s .

0 0

The motion i n this p r o b l e m m u s t be c o m p l e t e l y bounded. Since the t e r m i n equation (3.38a) which i s l i n e a r i n i s unbounded, i t m u s t be e l i m i n a t e d by r e q u i r i n g t h a t

F r o m the i n i t i a l conditions (3.14), g o = 0

A = O 0

15

B

2 - 1

/

2

0 8 e O a ~

3.8 C o m p a r i s o n of the Results with R e f e r e n c e 2

--

-

The final r e s u l t s m a y then be s u m m a r i z e d a s follows:

-

2 + 1 2 3

*

-

1 c o s ( l t ~ a ~ _{4 e a. )q - e o}

-t c o s

--

3

1

The corresponding r e s u l t s obtained i n r e f e r e n c e 2 a r e given below

1

-

s(l) =

15

8 e ~ [cos a ~

-

$-cos(2pl-l)$]

1

2

2

~ [ e o ( l - e o ) s i n

t

=

-

1 cos 9-e

a. 1-e cos J,

+

c o s -

In o r d e r to c o m p a r e the above r e s u l t s with t h e solution d e r i v e d by the p r e s e n t approach, i t i s n e c e s s a r y t o e x p r e s s the quantities p

1

:]e

and

Jr

u s e d i n equations (3.43) i n t e r m s of cp

.

The angle

IJI

was defined i n r e f e r e n c e 2 a s

2

Jr

= ( l t e wl)@ e3.44)

When the e x p r e s s i o n f o r

@

given by equation t 3 . 8 ~ ) together with v a l u e s of w

1 $

hl, and v given i n r e f e r e n c e 2 a r e used, equation (3.44) becomes: 1

It i s e a s y t o v e r i f y that t = t(0)+etil) can be expanded i n the f o r m :

Hence

Jr

b e c o m e s

R e f e r e n c e 2 a l s o g i v e s 3

s o that

In the above, i t i s c o n s i s t e n t t o neglect t e r m s of o r d e r e s i n c e ~ ( l ) and 0

t'l) a r e multiplied by e O .

i s e x a c t t o t h e o r d e r s r e t a i n e d , It should a l s o b e pointed out t h a t the r e s u l t s a r e i n exact a g r e e m e n t with the c l a s s i c a l l u n a r t h e o r y of ~ e ~ o n t e / c o u l a n t (c.f., r e f e r e n c e 3 and c o m m e n t s i n r e f e r e n c e 2).

3.9

S u m m a r y

In the method of r e f e r e n c e 2, t h e t e r m s of o r d e r c w e r e difficult t o d e r i v e and a r o s e somewhat a r t i f i c i a l l y a s the r e s p o n s e to a n a l m o s t r e s o n a n t forcing function i n the differential equations. In the p r e s e n t f o r m u l a t i o n t h e s e t e r m s a p p e a r quite n a t u r a l l y and no difficulties a r e a s s o c i a t e d with t h e i r evaluation.

F u r t h e r m o r e , the a p p r o a c h of r e f e r e n c e 2 f a i l s t o give a u n i f o r m l y valid approximation when applied t o o r b i t s with high

REFERENCES

1. Kevorkian, J., The Uniformly Valid Asymptotic R e p r e s e n - tation of the Solutions of C e r t a i n Non-Linear D i f f e r e n t i a l Equations, Ph. D , t h e s i s , Guggenheim A e r o n a u t i c a l L a b o r a t o r y , California Institute of Technology, 196 1.

2 , Kevorkian, J.

,

Uniformly Valid Asymptotic R e p r e s e n t a t i o n f o r All T i m e s of the Motion of a Satellite i n t h e Vicinity of the S m a l l e r Body i n the R e s t r i c t e d T h r e e - B o d y P r o b l e m , t o be published i n the A s t r o n o m i c a l J o u r n a l , May 1962.