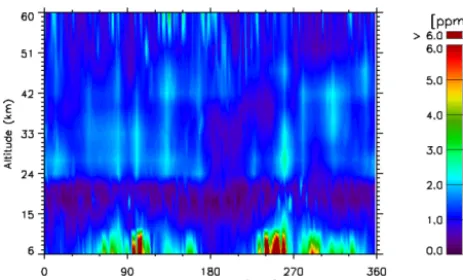
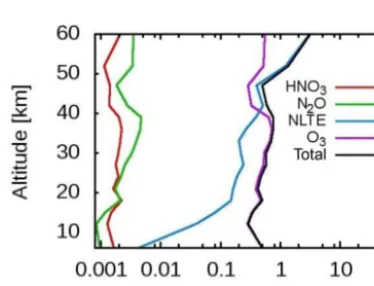
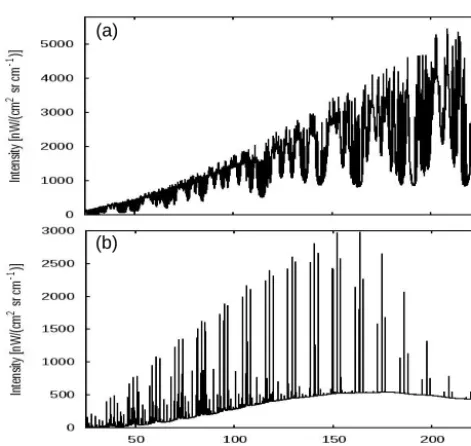
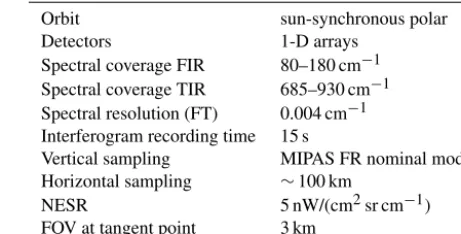
A strategy for the measurement of CO 2 distribution in the stratosphere
Full text
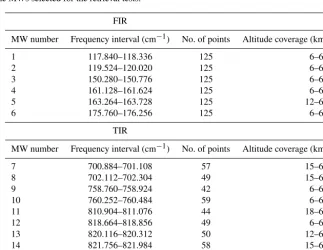
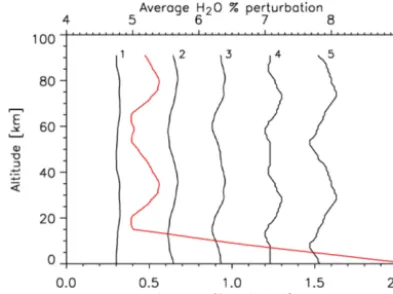
Figure
Related documents
• Follow up with your employer each reporting period to ensure your hours are reported on a regular basis?. • Discuss your progress with
Results suggest that the probability of under-educated employment is higher among low skilled recent migrants and that the over-education risk is higher among high skilled
In this PhD thesis new organic NIR materials (both π-conjugated polymers and small molecules) based on α,β-unsubstituted meso-positioning thienyl BODIPY have been
National Conference on Technical Vocational Education, Training and Skills Development: A Roadmap for Empowerment (Dec. 2008): Ministry of Human Resource Development, Department
Field experiments were conducted at Ebonyi State University Research Farm during 2009 and 2010 farming seasons to evaluate the effect of intercropping maize with
In this present study, antidepressant activity and antinociceptive effects of escitalopram (ESC, 40 mg/kg) have been studied in forced swim test, tail suspension test, hot plate